Page 3 - PDF_Flip_Book

Rod Machado’s Private/Commercial Pilot Handbook
2-14

The Lift Equation

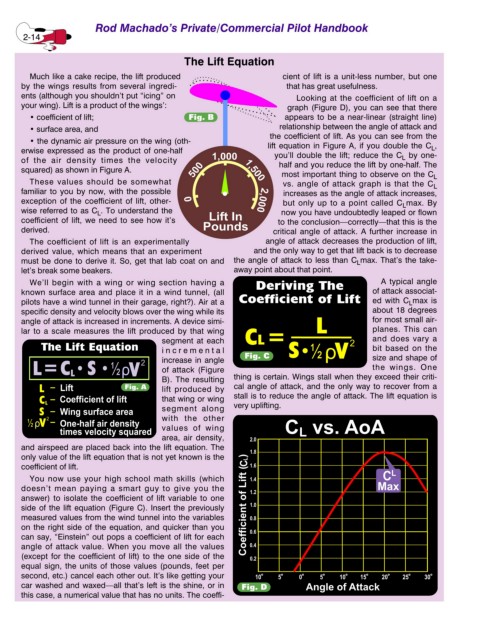
Much like a cake recipe, the lift produced cient of lift is a unit-less number, but one
by the wings results from several ingredi- that has great usefulness.
ents (although you shouldn’t put “icing” on Looking at the coefficient of lift on a
your wing). Lift is a product of the wings’: graph (Figure D), you can see that there
• coefficient of lift; Fig. B appears to be a near-linear (straight line)
• surface area, and relationship between the angle of attack and
the coefficient of lift. As you can see from the
• the dynamic air pressure on the wing (oth-
lift equation in Figure A, if you double the C ,
erwise expressed as the product of one-half L
you’ll double the lift; reduce the C by one-
of the air density times the velocity L
half and you reduce the lift by one-half. The
squared) as shown in Figure A.
most important thing to observe on the C
L
These values should be somewhat vs. angle of attack graph is that the C
L
familiar to you by now, with the possible increases as the angle of attack increases,
exception of the coefficient of lift, other- but only up to a point called C max. By
L
wise referred to as C . To understand the now you have undoubtedly leaped or flown
L
coefficient of lift, we need to see how it’s to the conclusion—correctly—that this is the
derived. critical angle of attack. A further increase in
The coefficient of lift is an experimentally angle of attack decreases the production of lift,
derived value, which means that an experiment and the only way to get that lift back is to decrease
must be done to derive it. So, get that lab coat on and the angle of attack to less than C max. That’s the take-
L
let’s break some beakers. away point about that point.
We’ll begin with a wing or wing section having a A typical angle
known surface area and place it in a wind tunnel, (all Deriving The of attack associat-
ed with C max is
pilots have a wind tunnel in their garage, right?). Air at a Coefficient of Lift L
specific density and velocity blows over the wing while its about 18 degrees
angle of attack is increased in increments. A device simi- for most small air-
lar to a scale measures the lift produced by that wing L planes. This can
and does vary a
=
segment at each CL
incremental Fig. C ρ
The Lift Equation bit based on the
increase in angle S V size and shape of
ρ of attack (Figure the wings. One
L=CL S
B). The resulting
V thing is certain. Wings stall when they exceed their criti-
Lift Fig. A lift produced by cal angle of attack, and the only way to recover from a
L
stall is to reduce the angle of attack. The lift equation is
Coefficient of lift that wing or wing
C L
Wing surface area segment along very uplifting.
with the other
S
ρ One-half air density
V values of wing C vs. AoA
L
times velocity squared L
area, air density, 2.0
and airspeed are placed back into the lift equation. The
1.8
only value of the lift equation that is not yet known is the L 1.6 CL
coefficient of lift.
You now use your high school math skills (which 1.4 C L
doesn’t mean paying a smart guy to give you the 1.2 Max
answer) to isolate the coefficient of lift variable to one
side of the lift equation (Figure C). Insert the previously Coefficient of Lift ( ) 1.0
measured values from the wind tunnel into the variables 0.8
on the right side of the equation, and quicker than you 0.6
can say, “Einstein” out pops a coefficient of lift for each
angle of attack value. When you move all the values 0.4
(except for the coefficient of lift) to the one side of the 0.2
equal sign, the units of those values (pounds, feet per
second, etc.) cancel each other out. It’s like getting your 10 5 0 5 10 15 20 25 30
car washed and waxed—all that’s left is the shine, or in Fig. D Angle of Attack
this case, a numerical value that has no units. The coeffi-